3 - The identification of the third point of nothing
The next two-oneness offers again two -and no more than two- possibilities to identify the next point of nothing: it can be chosen to be on the D1- line of nothing, or it can be chosen not to be on that D1- line.
The first possibility identifies the sizeless point P3 of nothing on the D1- line of nothing, but this also shows how the identity of P3 is not identical to the identity of P1 and P2, because only these two did define the unique & unambiguous D1- line in perfect accordance with all oerconditions, also quantisizing the unity of D1- distance. This means that P3 as chosen to be on that D1- line, must get a different symbol, here chosen to be an “open dot”, its symbol being “ 3 ”, the third “natural (counting) number”.
There is also another two-oneness offering two -and no more than two- possibilities: either a new unity of distance can be chosen. either the unity of distance remains the same or a new one is chosen, subjected to the oercondition that it must be longer than Ð1.
But all points of nothing which are located on that boundless, unlimited and infinite long D1- line disclose also one other, unique & unambiguous characteristic: each point of nothing has two -and no more than two- “sides”. The consequence is that P3 can be located at the same side of P1 as P2, or it can be chosen at the other side of P1, as shown by symbol P3’, P three dash.
This two-oneness turns out to be important when you realize that the process of creation
will not be different if our ancestors would have inverted symbol 1 and 2: once one “side” is chosen this choice must be continued: “there never will be a two-oneness which will offer access to the other side”...

3.1- The D1- line of natural (counting) numbers is
announcing the first operation in mathematics
It is important to realize that -right now- the Natural Start of the Beginning with Nothing shows no other dimension, characteristic, identity or entity etc. etc. than the first (oer-)dimension and its inseparable relation with natural (counting) numbers. This not only identifies D0- points of nothing on that D1- line of nothing but also quantisizes the distance or length between them.
Unfortunately it is not possible to arrive at this moment at a correct definition were
simple alpha-language will also show the relation with the other operations and
their beta-symbols. This will only be possible after all operations are identified as
will be shown.
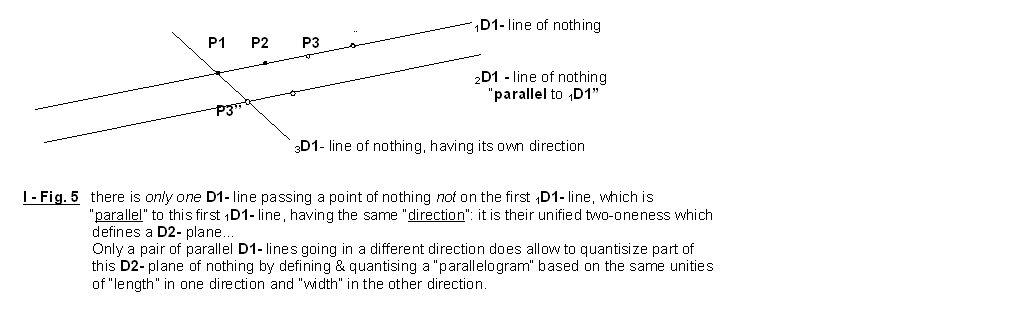
The second possibility identifies “the third point of nothing not on the 1D1- line of nothing”,
symbolised as P3’’, P three, double dash, or by any other unique symbol. This point also defines three D1- lines passing P1, P2 and P3 on the 1D1- line, and when more points of nothing are identified on this 1D1- line, there will be more lines as well: even a boundless, unlimited and infinite quantity... hence the oerconditions command:
one unique & unambiguous D1- line which is passing P3’’,
having no point of nothing in common with the 1D1- line of nothing
This is the famous fifth axiom of Euclid’s “parallel lines”, causing headaches in mathematics ever since, leading to fundamental deviations in Riemann's Non-Euclidean Geometry and hence in quantum-mechanics etc...
So far there are different possibilities to define a boundless, unlimited and infinite large D2- plane:
a - by three D0- points of nothing,
b - by a combination of one D0- point of nothing and a D1- line of nothing, since two
D0- points of nothing define one D1- line,
c - by two intersecting D1- lines of nothing, sharing one D0- point of nothing (similar to a).
And when more points of nothing are identified on this 1D1- line, there will be more
lines as well, actually a boundless, unlimited and infinite quantity.
These methods to define a D2- plane of nothing must now be replaced by the unique
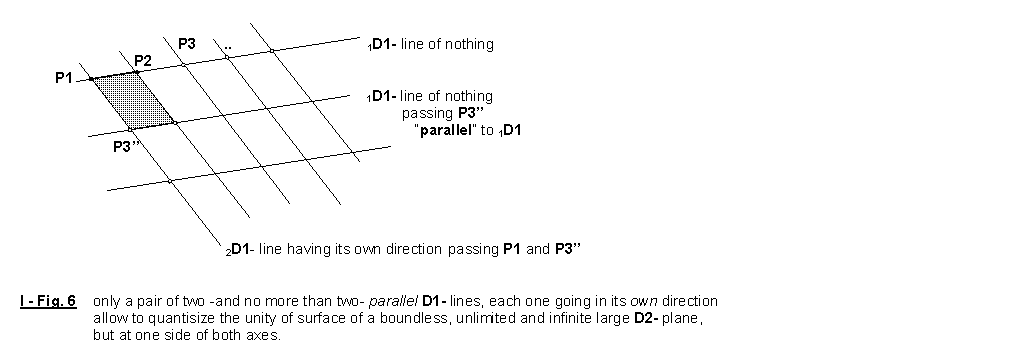
two-oneness of two -and no more than two- pair of “parallel” D1- lines which allow to quantisize part of this boundless, unlimited and infinite large D2- plane of nothing, being just as massless as D1- lines or points of nothing.
But there is more to discover: each D1- line in a D2- plane has a new unique & unambiguous characteristic, called its “direction”, hence only “parallel” D1- lines do have the same direction.
And although this is in perfect accordance with the oerconditions, there is no method to define & quantisize “directions of D1- lines” in an objective way... ordering you to wait till the logistic order of the process of creation will disclose such objective method.
Now a D2- plane is defined in accordance with all oerconditions as boundless, unlimited and infinite large plane, its unity of “surface” must be "quantisized”. Since each D1- line which unifies P3’’ not on the first 1D1- line of nothing with each point P on that first 1D1- line of nothing, the third 3D1- line which is passing P1 and P3’’ has another direction.
This other direction shows how one other D1- line of nothing which is passing P2 and which is parallel to this 3D1- line of nothing also allows to quantisze the unity of
D2- surface of this D2- plane:
There is indeed an absolute freedom to chose the unity on the second directio to be different from the chosen unity of length in the first direction as shown by the distinction between "length" in the first direction and "breadth or width" in the other direction, but the habit to state that length is always longer than width" is now overruled by the definition of Đ1 as smallest possible unity of distance or length in the process of creation.
But different unities of distance or length in different directions are leading to ambiguities, even when the initial 1D1- line with its growing quantity of natural (counting) numbers will
be renamed as “X- axis” and the 3D1- line of nothing which is passing P1 and P3’’ will be the “Y- axis”, especially when the sequence of these letters in the alphabet suggests that X always comes first...
As mentioned before it is important to realize and accept that there is no objective method to define & quantisize the direction of a D1- line in a D2- plane...
and only after the first two oerdimensions of the cycle of the process are unified, you will discover their secret and understand why there never will be an objective method.
3.2 - Symmetry is unifying
Now a D1- line parallel to the X- axis and a D1- line parallel to the Y- axis define a parallelogram as unity of surface which allows to quantisize part of a boundless, unlimited and infinite large D2- plane, Euclid’s geometry identifies four special points of nothing as “edges, corners or vertex” (plural: vertices) and two pairs of “sides”, referring to parts of D1- lines which are enclosing this parallelogram. When each side of the parallelogram has the same D1- length, it is said to be “regular”, its alpha-name being a “rhombus”. And because there is no objective method to define & quantisize the direction of a D1- line in an objective way, the direction of the X- axis is taken as subjective reference, but this discloses also how there is a boundless, unlimited and infinite quantity of directions of the
Y- axis, hence there must be one unique & unambiguous direction which is “perpendicular” to the X- axis and whatever direction is taken first, they are always “perpendicular to each other”.
When the sides of a rhombus are perpendicular to each other, this is a “square” and when opposed vertices are connected by D1- lines, these “diagonals” are also perpendicular to each other, having the same length, their intersection being a special “central” point of nothing: the “point of symmetry” in all directions.
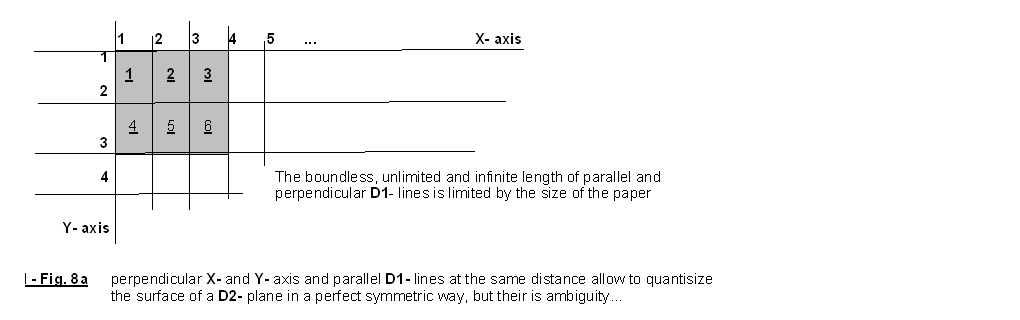
The alpha-word “perpendicular” also means that the “projections” of all points of nothing on that D1- line, a boundless, unlimited and infinite quantity, on the other D1- line reduce to just “the same point of nothing: the point of intersection”. Next figure shows two X- and Y- axis which are “perpendicular” to each other, when now the unity of distance between two -and no more than two- successive natural (counting) numbers is the same, the unity of surface of their D2- plane is a “square”, the smallest possible one based on Ð1.
But even when the unity of D1- distance or length in X- direction is equal to the unity of “width” in Y- direction, using the same natural (counting) numbers will confirm that a quantity of n. D1- distances is not same as a quantity of n. D2- squares. And even when by convention “length will be longer than width” there is another problem as well...
3.3 - Quantisizing parts of a D2- plane forces to identify
an undeniable non- natural (counting) number
Last figure reveals a fundamental problem, showing again the inconsistency between alpha α-words and beta β-signs... For example: number 3 on the X- axis defines just two unities of distance or length, and number 4 on the Y- axis defines just three unities of distance or width, even when unities are the same. And although there is only one common point on the X- axis and Y- axis, it does have two numbers 1...
Around 500-800 CE the solution was found in Babylon when the need for an unambiguous administration in trade forced also to count empty storage rooms: these were indicated by their alpha-name “sunya” its symbol " O " enclosing its void. Becoming generally accepted in trade and commerce, the application of this “non-natural” (counting) number in mathematics was expanding very slowly, getting the name "zero" in the West. Next figure shows how each natural (counting) number on each axis is translated over one unity of distance, the open space unifying alpha α- words and beta β- signs: only now beta-sign 3 which did identify point 3 by that number is consistent with the alpha word “three” defining & quantisizing three unities of D1- distance or length to the local origin O, on whatever axis, going in whatever direction.

Only now the first mathematical operation of “unifying by adding” is showing the unification between α- language and the β- formula: when for example 2 unities of distance or length are “added” to 3 unities on that same X- axis// D1- line, the beta-symbol “ + ” instructs to unify 2 + 3 as D1- distance between point P0 to point P2 + (plus) the distance between point P0 to point P3, unified as one distance between P0 and P5, written in β- symbols: 0 to 2 + 0 to 3 = 0 to 5 unities of D1- distance. But when all zeros at both sides of the = sign are left out, this is shortened to 2 + 3 = 5. And when is started with the other term, this proves that the unification of 3 + 2 will give the same unique & unambiguous result.
Multiplying as second operation in mathematics
Counting unities of D2- surface now reveals that it is just a “repeating addition in the second direction” the example shows a first row of 3 unities in X- direction which is completed by the second row, counting the underlined number 4 , 5 and 6 the initial instruction “ + ” to unify by adding is now rotated in an “ x “ to “unify by multiplying” hence there are 2 x 3 = 6 squares, allowing you to observe how alpha-language speaks of two “times” three, another indication that something has been lost...
Starting in the other Y- direction shows three rows of two, in algebra this is called
“commutative” because 1 + 2 = 2 + 1 and 2 x 3 = 3 x 2 = 6, actually denying & darkmooning the impossibility to define & quantisize a direction of a D1- line in a
D2- plane in an objective way...
When -much later in the process of creation- other possibilities, dimensions,
characteristics, identities or entities etc. etc. will be identified, each unity can always
be represented or symbolised by a unity of D1- distance or length, hence the first
mathematical operation of “unifying by adding” is inseparably related to the
identification of sizeless points of nothing on the D1- line of nothing by natural
(counting) numbers and some chosen D1- distance between two points of nothing,
the alpha word “successive” referring to the two-oneness of P1 and P2...
Now only points of nothing on this first 1D1- line of natural (counting) numbers are identified by their own unique & unambiguous natural (counting) number when the
D1- distance between each successive pair is the same, the new number “sunya or zero” is special: does each point Px disclose to have two -and no more than two- “sides”: one side facing “higher numbers” being perfectly opposed to its other side which is facing “lower numbers”, this is not valid for number sunya or zero... This zero, 0 also has two -and no more than two- sides, but only one side is facing the part of a D1- line with natural (counting) numbers whereas its other side is facing the part with no natural (counting) numbers, making sunya or zero known as "non-natural (counting) number”. And just as it turned out to be impossible to define & quantisize the direction of a D1- line in a D2- plane in an objective way, it is impossible to identify a two-oneness which would give access to the other side of sunya or zero, its “negative” side, a conclusion which will have serious consequences. And only much later after the start with nothing, you can enjoy your own unique & absolute unambiguous image in a flat D2- plane of a mirror...
.
.